You must log in or register to comment.
It’s possible to draw anything with a math function?
Short answer yes
But it actually depends on what you mean by draw
With polar coordinates and Fourier transforms, you can draw the outlines of tons of figures. But you can’t go back. Imagine that you can go with your pen around the center in only one direction, but any distance from the center.
… I think you can go back… but maybe I didn’t get your explanation
You could draw a representation of anything with a math function, yes. Have fun calculating anything complex though.
I love that but it’s missing 2 leaves
I got two more leaves for you 🥦
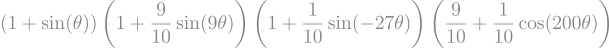
The number of leaves depends on the age of the plant. There are ones with one and three leaves as well. However I’ve never seen a leaf with a bud in the middle like on the left.
It’s a mutation, happens rarely. I’ve had a couple of plants do it in the 5 or so years I’ve been growing.
A web search tells me the θ (lower-case theta) is used to represent an angle. Do you just fill in 0° – 359.9° one after another to draw that curve?
Yes. https://en.m.wikipedia.org/wiki/Polar_coordinate_system
Since this curve is cyclical, you can do it [-infinity ; +infinity] and it’s the same curve again and again.
Thanks. 🙂
I like how you have all those random-ass coefficients and then there’s just 1+sinθ chilling there.
It’s basically the length of the leaves. Wonderful how this can be described so simply!
